Abstract
Few methods for demonstrating the effects of species interactions rival that of the manipulative experiment (Kareiva and Levin 2002). The now commonly performed removal or addition of predators, competitors, or mutualists to experimentally replicated populations of recipient species has irrefutably shown that species can have important effects on each other’s populations, and that the strengths of these effects can vary considerably across environmental contexts and species identities. A large body of research is directed towards understanding this variation to forecast community dynamics and dissect how species interactions regulate community structure (Chesson 2000, McCann 2000, Stachowicz 2001, Duffy 2002). It has frequently been pointed out that progress in this field will require more explicit connections between ecological theory and the realities of nature (Laska and Wootton 1998, Parker et al. 1999, Berlow et al. 2004, Agrawal et al. 2007). This requires that interaction strengths measured experimentally be appropriate to the biology of our study systems and the mathematical abstractions we ascribe to them.
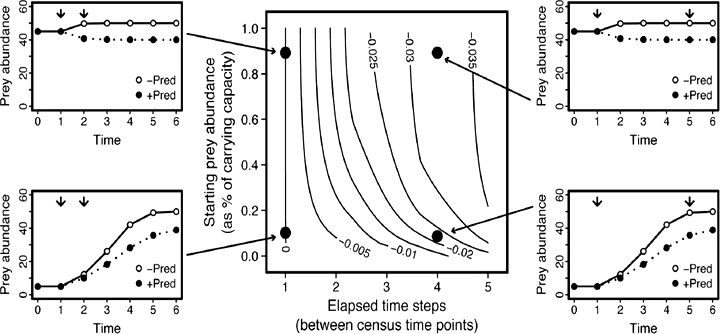
Here we clarify some of the assumptions made in the application of the two most commonly used indices for measuring the strengths of species interactions with manipulative removal/addition experiments: Paine’s index and the dynamic index. We explain how the values these indices are intended to measure – the per capita interaction strength between two species – are typically not estimated in common currencies. We then introduce extensions to these indices that alleviate a subset of previously made assumptions and limitations. These include a reformulation of Paine’s index appropriate for an open‐recruitment system, and an extension of the dynamic index applicable to interactions of a particular nonlinear form. While we focus our language on predator–prey interactions, our discussions are pertinent to the measurement of species effects in other types of interactions as well (Mitchell and Wass 1996, Freckleton et al. 2009).